Find the length of the third side. If necessary, write in simplest radical form.

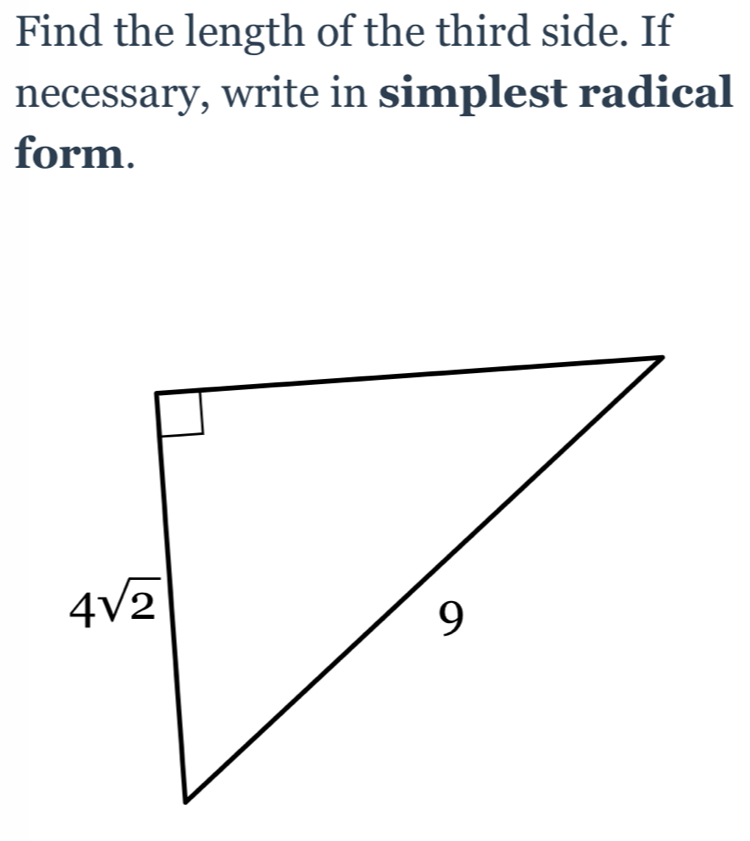
Answer:[tex]\boxed {\boxed {\sf 8}}[/tex]Step-by-step explanation:This triangle has a small square, which represents a right angle. Therefore, we can use the Pythagorean Theorem. [tex]a^2+b^2=c^2[/tex]Where a and b are the legs of the triangle and c is the hypotenuse. In this triangle, 7 and √15 are the legs, because these sides make up the right angle. The unknown side is the hypotenuse, because it is opposite the right angle. So, we know two values: [tex]a= 7 \\b= \sqrt{15}[/tex]Substitute these values into the formula. [tex](7)^2+(\sqrt{15})^2=c^2[/tex]Solve the exponents. (7)²= 7*7=49 [tex]49+ (\sqrt{15})^2=c^2[/tex](√15)²=√15*√15=15[tex]49+15=c^2[/tex]Add.[tex]64=c^2[/tex]Since we are solving for c, we must isolate the variable. It is being squared and the inverse of a square is the square root. Take the square root of both sides. [tex]\sqrt{64}=\sqrt{c^2} \\\sqrt{64}= c\\8=c[/tex]The third side length is 8.